Curl mathematics wikipedia
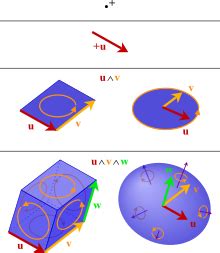
WebThe curl of a vector field is a vector function, with each point corresponding to the infinitesimal rotation of the original vector field at said point, with the direction of the … In vector calculus, the curl is a vector operator that describes the infinitesimal circulation of a vector field in three-dimensional Euclidean space. The curl at a point in the field is represented by a vector whose length and direction denote the magnitude and axis of the maximum circulation. The curl of a field is formally … See more The curl of a vector field F, denoted by curl F, or $${\displaystyle \nabla \times \mathbf {F} }$$, or rot F, is an operator that maps C functions in R to C functions in R , and in particular, it maps continuously differentiable … See more Example 1 The vector field can be … See more The vector calculus operations of grad, curl, and div are most easily generalized in the context of differential forms, which involves a number of steps. In short, they correspond to the derivatives of 0-forms, 1-forms, and 2-forms, respectively. The geometric … See more • Helmholtz decomposition • Del in cylindrical and spherical coordinates • Vorticity See more In practice, the two coordinate-free definitions described above are rarely used because in virtually all cases, the curl operator can be applied using some set of curvilinear coordinates, … See more In general curvilinear coordinates (not only in Cartesian coordinates), the curl of a cross product of vector fields v and F can be shown to be See more In the case where the divergence of a vector field V is zero, a vector field W exists such that V = curl(W). This is why the magnetic field, characterized by zero divergence, can be expressed as the curl of a magnetic vector potential. If W is a vector field … See more
Curl mathematics wikipedia
Did you know?
WebIn vector calculus, a conservative vector field is a vector field that is the gradient of some function. A conservative vector field has the property that its line integral is path independent; the choice of any path between two points does not change the value of the line integral. Path independence of the line integral is equivalent to the vector field under … WebIn vector calculus, the curl is a vector operator that describes the infinitesimal rotation of a vector field in three-dimensional Euclidean space. At every point in the field, the curl of that point is represented by a vector. Given a vector field , the curl of can be written ...
WebMathematically, the vorticity is defined as the curl (or rotational) of the velocity field of the fluid, usually denoted by and expressed by the vector analysis formula , where is the nabla operator and is the local flow velocity. [5] http://dictionary.sensagent.com/Curl%20(mathematics)/en-en/
WebThe curl at a point in the field is represented by a vector whose length and direction denote the magnitude and axis of the maximum circulation. The curl of a field is formally defined … WebMathematically, the vorticity of a three-dimensional flow is a pseudovector field, usually denoted by , defined as the curl of the velocity field describing the continuum motion. In Cartesian coordinates : In words, the vorticity tells how the velocity vector changes when one moves by an infinitesimal distance in a direction perpendicular to it.
WebIt is frequently helpful in mathematics to refer to the elements of an array using subscripts. The subscripts can be integersor variables. The array takes the form of tensorsin general, since these can be treated as multi-dimensional arrays. Special (and more familiar) cases are vectors(1d arrays) and matrices(2d arrays).
WebCurl (mathematics) synonyms, Curl (mathematics) pronunciation, Curl (mathematics) translation, English dictionary definition of Curl (mathematics). v. curled , curl·ing , curls … simplifi reviews 2022Web: it is the angle between the z -axis and the radial vector connecting the origin to the point in question. The azimuthal angle is denoted by : it is the angle between the x -axis and the projection of the radial vector onto the xy -plane. raymond ouryWebMath S21a: Multivariable calculus Oliver Knill, Summer 2011 Lecture 22: Curl and Divergence We have seen the curl in two dimensions: curl(F) = Q x − P y. By Greens theorem, it had been the average work of the field done along a small circle of radius r around the point in the limit when the radius of the circle goes to zero. raymond overcoatWebMar 24, 2024 · (1) where the surface integral gives the value of integrated over a closed infinitesimal boundary surface surrounding a volume element , which is taken to size zero using a limiting process. The divergence of a vector field is therefore a scalar field. If , then the field is said to be a divergenceless field. raymond outlook loginWebFrom Wikipedia, the free encyclopedia In vector calculus, the curl is a vector operator that describes the infinitesimal rotation of a 3-dimensional vector field. At every point in the … raymond overmanWebthe ∇× symbol (pronounced "del cross") denotes the curl operator. Integral equations [ edit] In the integral equations, Ω is any volume with closed boundary surface ∂Ω, and Σ is any surface with closed boundary curve ∂Σ, The equations are a little easier to interpret with time-independent surfaces and volumes. simplifi veriform pharmacyWebAs the name implies the curl is a measure of how much nearby vectors tend in a circular direction. In Einstein notation, the vector field has curl given by: where = ±1 or 0 is the Levi-Civita parity symbol . Laplacian [ edit] Main … simplifi transfer to investment account